We’re now shifting gears, and deviating from the syllabus, to touch on a classic topic that will set the stage for our Fluid Friday discussions this Friday.
A fantastic book I’ve recently discovered covering the last half of class is “How fluids unmix: Discoveries by the School of Van der Waals and Kamerlingh Onnes” by Johanna Levelt-Sengers. The book covers the primary historical context from which we can understand the status quo. Levelt-Sengers studies in Kamerlingh Onnes’ lab under Michels and is very familiar with the historical background.
The real triumph of the Van der Waals equation started from the very beginning. Van der Waals wrote his equation
(P+a/V^2)(V-b)=RT
and realized he could find the critical point from derivatives: (∂P/∂V)|T=0, (∂²P/∂V²)|T=0:
By solving for the critical parameters directly we get:
Pc=a/27b², Vc=3b, RTc=8a/(27b), and the combined critical ratio of PcVc/RTc=3/8.
This then allowed his equation to be generalized to other fluids if the critical parameters themselves are known. Know Pc, Vc, and Tc? Then you can find a and b for the fluid. Plug a and b into the top equation and you can P, V, or T.
Van der Waals propsed directly using the critical parameters as units of measurement in his equation. Mathematically:
(P*+3/V*²)(3V*-1)=8T* with P*=P/Pc, V*=V/Vc, T*=T/Tc.
Note that this equations is generalized, or universal. The reduces pressures of two fluids are the same if the fluids are in corresponding states, that is, at the same reduced volume and temperature. Kamerlingh Onnes used this technique to estimate the amount of liquid hydrogen he would need to liquefy helium. He wrote Van der Waals a letter stating:
“In what I described to you, your theory has been my guide. To calculate the critical temperature of a permanent gas from the [P-V] isotherm brings your dissertation to memory in a new way. The calculations were performed entirely on the basis of the law of corresponding states. Guided by that law, I estimated – even though I did not put that on paper – to need 20 liters [of hydrogen]. Had I estimated a few liters fewer, the experiment would not have succeeded – had I estimated much more, then I would have judged it unwise to proceed, in view of the available resources.” ~ Letter from Kamerlingh Onnes to Van der Waals on his retirement
As demonstrated, Van der Waals’ equation is good enough to get in the ballpark, he used saturated vapor pressure (P,T) data of water to estimate the critical temperature of 390°C, not far from 373.9°C for an extrapolation of 150°C. However the inaccuracies of his cubic equation became clear, estimation of the critical ratio for common fluids ranged from 0.23 for water to 0.29 for nobel gases, far away from the 3/8 his equation predicted. As you know, we developed better cubic equations (Peng-Robinson and Redlich-Kwong) and corresponding states can be applied in much the same with these as Van der Waals originally intended. So in the end, the principle — of corresponding states — was established and is still used today.
Let’s apply this in an example. Remember back to our derivation of the virial coefficients when the compressibility (Z) originated? Compressibility, Z=Pv/(RT), is similar to the critical ratio above as Van der Waals originally defined. Now, 150 years later we have many experimentally determined corresponding fluid states to test the theory with. When taking most normal fluids it’s then possible to overlay different fluid measurements on the same compressibility plot similar to the following:
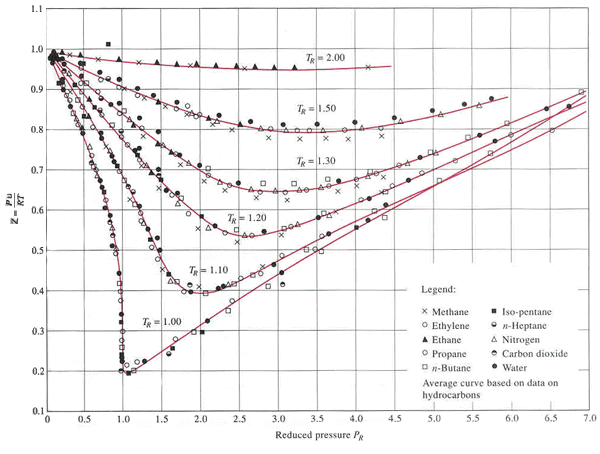
Reference: Cengel, Y. A. and Boles, M. A., “Thermodynamics: An Engineering Approach”, 7th ed., WCB/McGraw-Hill, Boston, 2011.
Let’s estimate the specific volume of nitrogen at T=150 K and P=8.5 MPa using the above chart. You’ll need the critical point information for N2: Tc= 126.19 K, Pc= 3395800 Pa, Vc= 1/313.3 m³/kg, and R=296.8 J/kg-K.
Now let’s do the same for hydrogen at T=40 K and P=3.2 MPa using the above chart. You’ll need the critical point information for H2: Tc=33.145 K, Pc=1296400 Pa, Vc=1/31.362 m³/kg, and R=4124 J/kg-K.
How’d you do?
Nitrogen: v_act=0.002834 [m^3/kg], Hydrogen: v_act=0.02441 [m^3/kg]